OS-V: 0530 Composite Shell Bending
This problem discusses about the composite shells two- or three-layer plate subjected to a sinusoidal distributed load, as described by Pagano (1969).
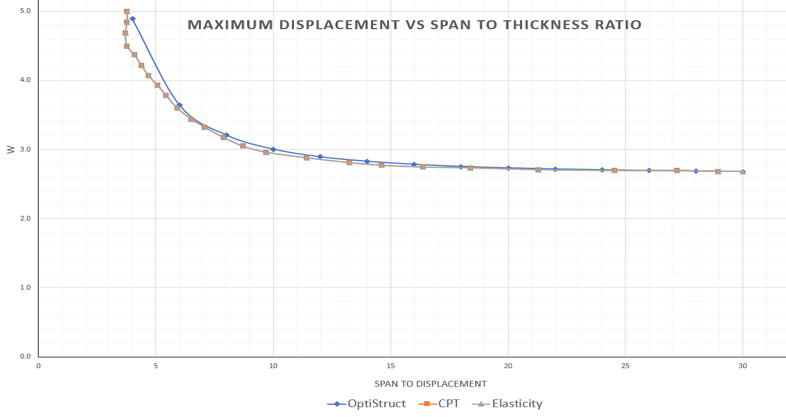
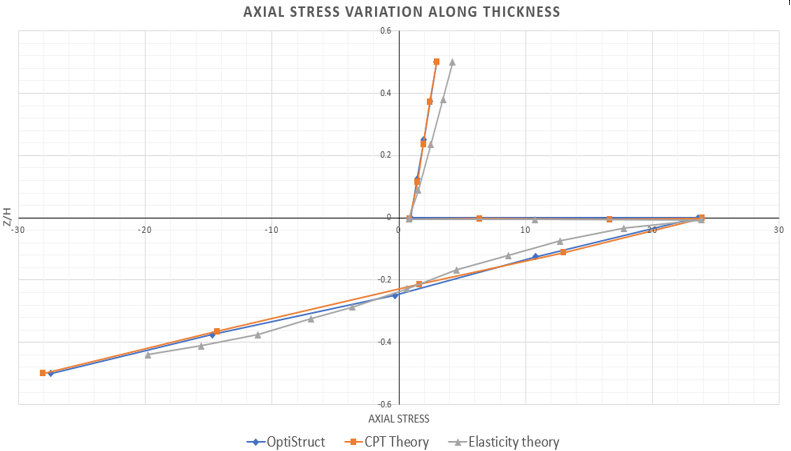
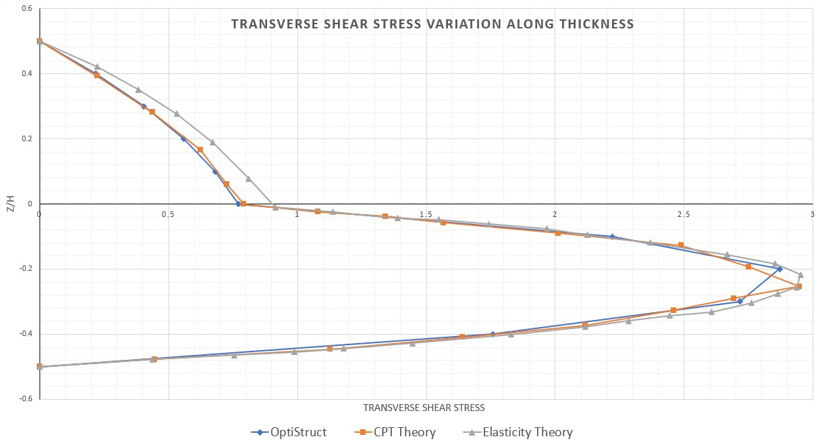
The resulting transverse shear and axial stresses through the thickness of the plate are compared to analytical solutions using classical laminated plate theory (CPT) and linear elasticity theory.
Benchmark Model
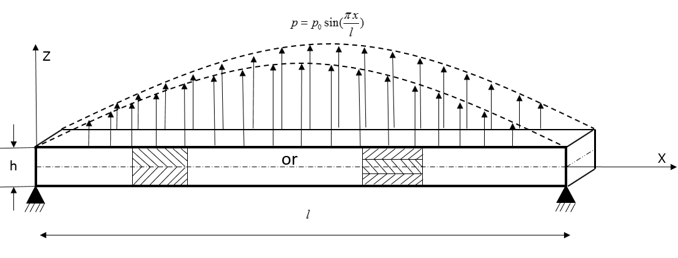
- For the two-layer model, top layer is in 90° and bottom layer is in 0°.
- For the three-layer model, the top and bottom layer are in 0° orientation and the middle ply is in 90° orientation.
- EL
- 25*106 lb/in2 (172.4 GPa)
- ET
- 1.0*106 lb/in2 (6.90 GPa)
- GLT
- 0.5*106 lb/in2 (3.45 GPa)
- GTT
- 0.2*106 lb/in2 (0.2 GPa)
- VLT = VTT
- 0.25
- L
- Signifies the direction parallel to the fibers
- T
- Signifies the transverse direction
| Stress Value | Xt | Xc | Yt | Yc | S |
|---|---|---|---|---|---|
| GPa | 2.07*10-4 | -8.28*10-5 | 3.45*10-6 | -1.03*10-5 | 6.89*10-6 |
| lb/in2 | 30.0 | -12.0 | 0.5 | -1.5 | 1.0 |
Results
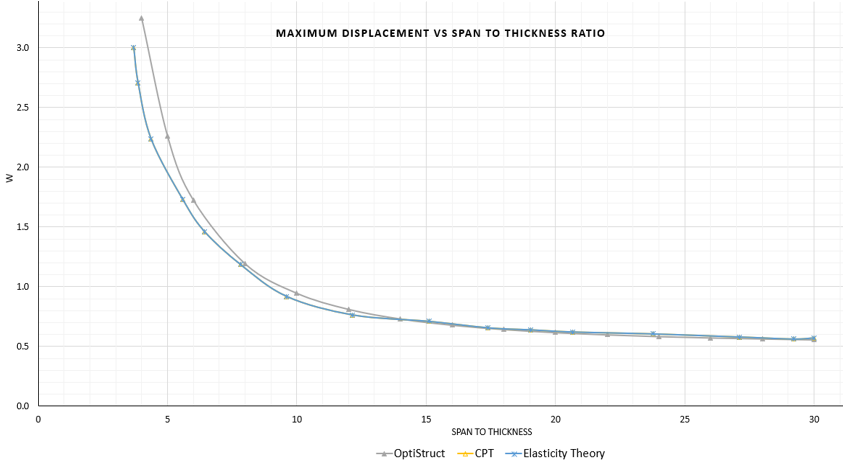
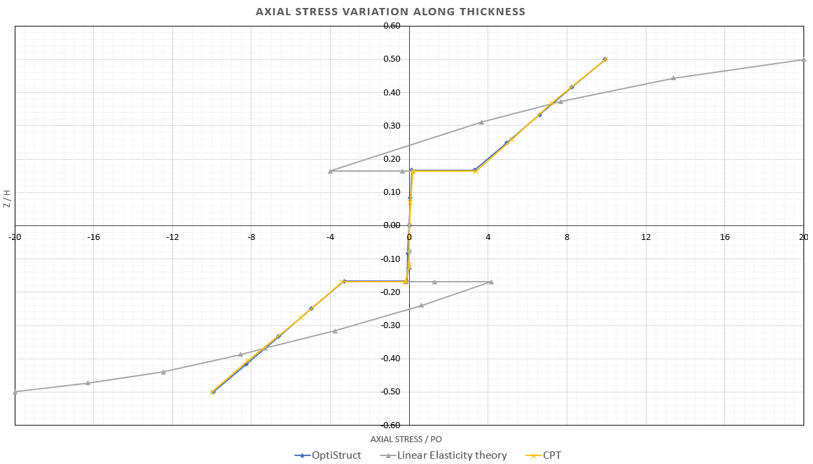
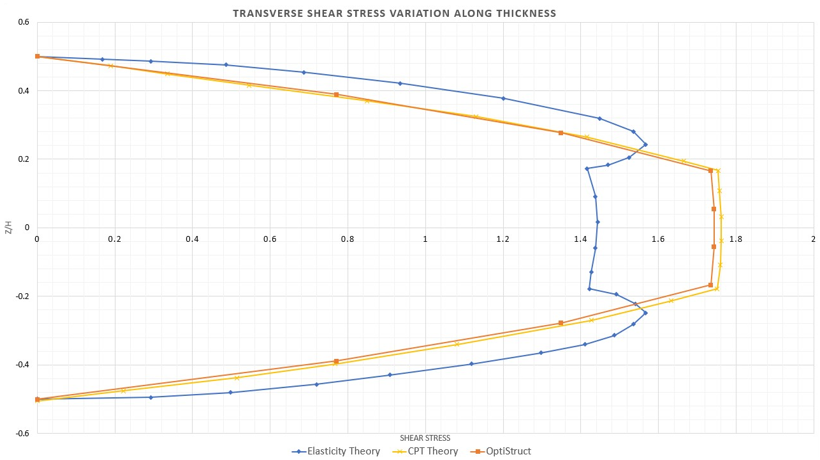
Model Files
The model files used in this problem include:
- /composite-shells-2-plys-2nd-order-span-4.fem
- /composite-shell-1st-order_span-4.fem